There are many techniques and synthesis styles out there, and we are truly living in the times where very little is impossible when it comes to sound alteration. The goal of this blog is to find as much information as I can. Today I would like to take a closer look at the quite simple, yet very effective type of synthesis called The Karplus-Strong Synthesis.
Developed and published in 1983 by Kevin Karplus and Alexander Strong, this algorithm has opened new doors for the possibilities in physical modelling. The sound was resembling in a very realistic way plucked string instruments such as guitar or banjo, and it
was initially named "digitar" synthesis. When it comes to the classification this technique falls into the wavetable category although one could argue in favour of subtractive synthesis as it relays on the feedback loop.
The idea is simple. All we need something to trigger a short impulse from a white noise generator, delay and band-pass filter. The white noise acts as an exciter in this example and was also used in the original algorithm. Delay time should be very quick around a few milliseconds so it creates very fast rattling effect at the audio rate speed. The faster the delay time the higher the pitch. By controlling the feedback of the delay we can increase or decrease sustain of our tone.
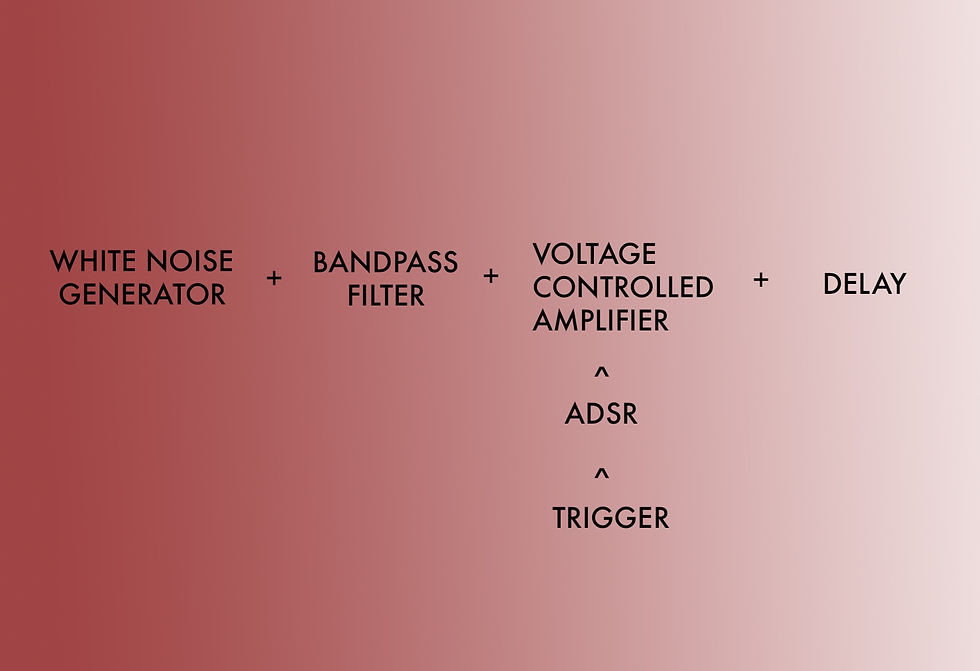
At this point, we will struggle to generate anything musically meaningful, that's because we need something to translate the delay time into the pitch. The fundamental of our sound will be represented in whole-number multiple of the sampling period and we need to say it in the language of chromatic tones. This can be solved with the interpolation filters.
This algorithm isn't limited only to generating pluck sounds, by carefully adjusting our band-pass filter and the noise generator envelop we can get more percussive (bongo, conga), woodwind or bowed string instruments.

The Karplus-Strong oscillators can be found in euro rack modules and also in Arturia's recent digital-hybrid synthesizer MicroFreak. The algorithm is now patented by Yamaha, and it seems that the actual inventors are not being credited enough, but hopefully this will change as we may see more hardware synths using this algorithm.
Stay Tuned
Jake

Comments